Morphological operations, part 1

Morphological image processing is a field of computer vision that deals with the removal of imperfections in binary and grayscale images.

ImageMorphology contains a set of non-linear operations related to the shape or morphology of image elements.

In this demonstration, we will look at 8 of the most common morphological operations.

Pkg.add(["TestImages", "ImageMorphology"])

using Images
using ImageMorphology, TestImages

img = Gray.(testimage("morphology_test_512"))

Erosion

The erode function performs minimal filtering on nearest neighbours. The default is 8 links in 2d, 27 links in 3d, etc. You can specify a list of dimensions you want to include in the connectivity, e.g. region = [1,2] to exclude the third dimension from filtering.

Below is an example of how erosion works on a binary image using a 3x3 structuring element.

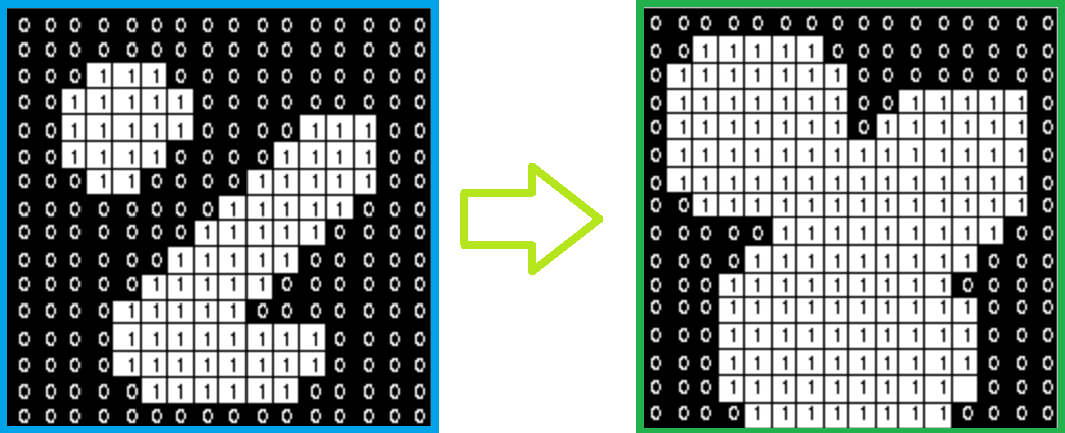
img_erode = @. Gray(img < 0.8); # keeps white objects white
img_erosion1 = erode(img_erode)
img_erosion2 = erode(erode(img_erode))
mosaicview(img_erode, img_erosion1, img_erosion2; nrow = 1)

Expansion

The dilate function performs maximising filtering on its nearest neighbours. By default it works the same way as erode.

img_dilate = @. Gray(img > 0.9);
img_dilate1 = dilate(img_dilate)
img_dilate2 = dilate(dilate(img_dilate))
mosaicview(img_dilate, img_dilate1, img_dilate2; nrow = 1)

Discovery

The morphology operation opening is equivalent to dilate(erode(img)). In opening(img, [region]), region allows you to control the dimensions over which this operation is performed. Opening can remove small bright spots and join small dark cracks.

img_opening = @. Gray(1 * img > 0.5);
img_opening1 = opening(img_opening)
img_opening2 = opening(opening(img_opening))
mosaicview(img_opening, img_opening1, img_opening2; nrow = 1)

Closing

The closing morphology operation is equivalent to erode(dilate(img)). The function closing(img, [region]) works similarly to opening. Closing can remove small dark spots and join small light coloured cracks.

img_closing = @. Gray(1 * img > 0.5);
img_closing1 = closing(img_closing)
img_closing2 = closing(closing(img_closing))
mosaicview(img_closing1, img_closing1, img_closing2; nrow = 1)

Top-hat

The tophat function is defined as the image minus its morphological opening. tophat(img, [region]) works similarly to opening and closing. This operation returns bright spots of the image that are smaller than the structuring element.

img_tophat = @. Gray(1 * img > 0.2);
img_tophat1 = tophat(img_tophat)
img_tophat2 = tophat(tophat(img_tophat))
mosaicview(img_tophat, img_tophat1, img_tophat2; nrow = 1)

Bottom-Hat

The Bottom-Hat morphology operation is defined as the morphological closure of an image minus the original image. Bothat(img, [region]) is defined similarly tophat. This operation returns the dark spots of the image that are smaller than the structuring element.

img_bothat = @. Gray(1 * img > 0.5);
img_bothat1 = bothat(img_tophat)
img_bothat2 = bothat(bothat(img_tophat))
img_bothat3 = bothat(bothat(bothat(img_tophat)))
mosaicview(img_bothat, img_bothat1, img_bothat2; nrow = 1)

Morphology gradient

The morphological gradient is the difference between the expansion and erosion of an image. morphogradient(img, [region]) is defined in the same way as tophat.

img_gray = @. Gray(0.8 * img > 0.7);
img_morphograd = morphogradient(@. Gray(0.8 * img > 0.4))
mosaicview(img_gray, img_morphograd; nrow = 1)

Laplace morphological operator

The morphological Laplace operator returns a value that is defined as the arithmetic difference between the inner and outer gradient. The function morpholaplace(img, [region]) is defined similarly tophat.

img_gray = @. Gray(0.8 * Gray.(img) > 0.7);
img_morpholap = morpholaplace(img_gray)
mosaicview(img_gray, img_morpholap; nrow = 1)

Conclusion

In this example, we have broken down some morphological operations that can be applied to images.