Engee documentation
Notebook

Modelling of LC-oscillator based on Colpitts scheme

This example shows the implementation of a Colpitts oscillator circuit with a nominal frequency of 9 MHz. The oscillation frequency is given by the formula:

Schematic diagram of the model:

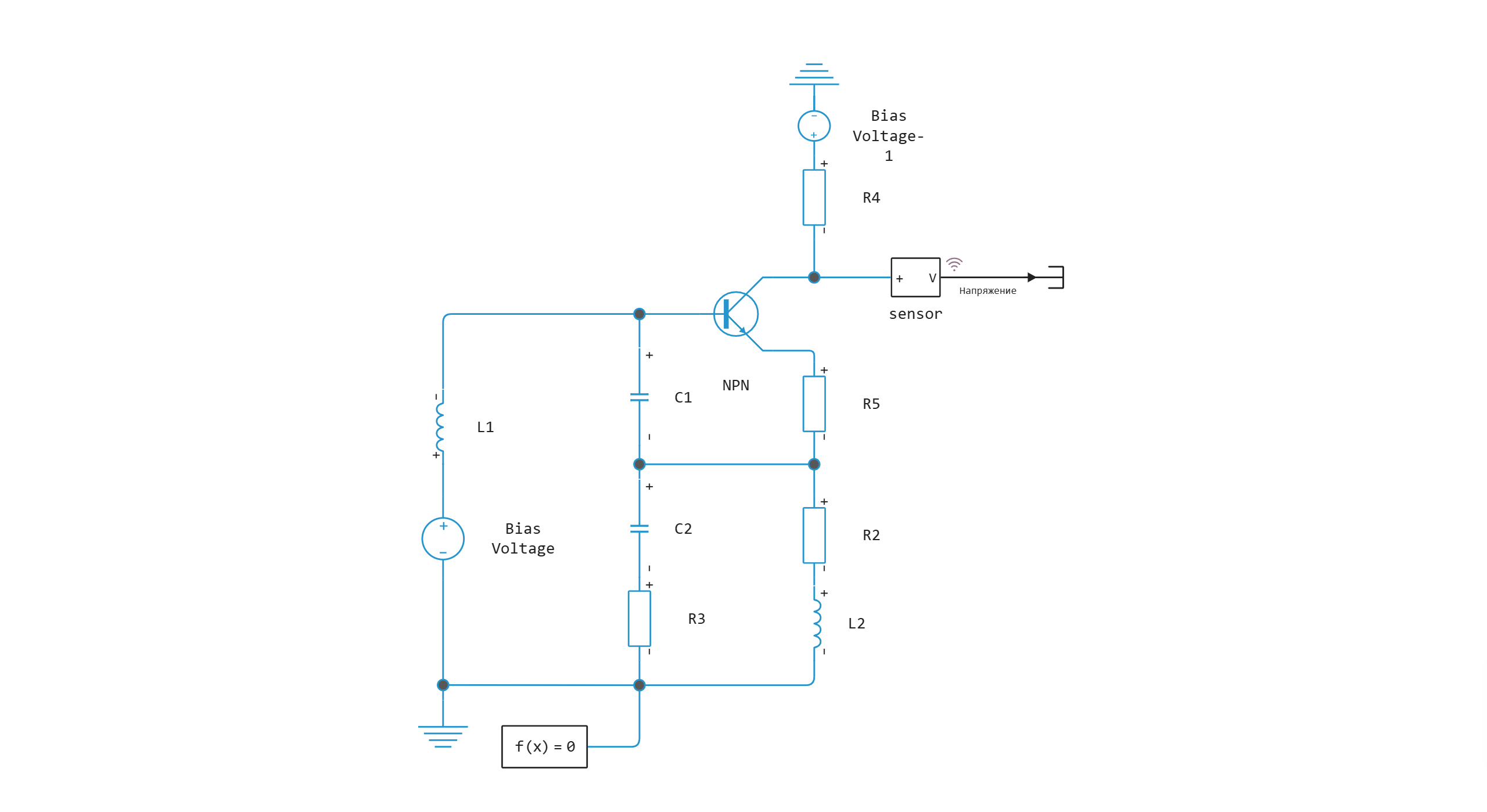
lc_oscillator--1734963351199.png

Define the function to load and run the model:

In [ ]: 
function start_model_engee()
    try
        engee.close("lc_oscillator", force=true) # закрытие модели 
        catch err # в случае, если нет модели, которую нужно закрыть и engee.close() не выполняется, то будет выполнена её загрузка после catch
            m = engee.load("$(@__DIR__)/lc_oscillator.engee") # загрузка модели
        end;

    try
        engee.run(m) # запуск модели
        catch err # в случае, если модель не загружена и engee.run() не выполняется, то будут выполнены две нижние строки после catch
            m = engee.load("$(@__DIR__)/lc_oscillator.engee") # загрузка модели
            engee.run(m) # запуск модели
        end
end
Out[0]:
start_model_engee (generic function with 1 method)

Running the simulation

In [ ]: 
try
    start_model_engee() # запуск симуляции с помощью специальной функции, реализованной выше
    catch err
    end;

Write from simout to voltage signal variables:

In [ ]: 
res = collect(simout)
V = collect(res[1])

Visualisation of simulation results

In [ ]: 
using Plots
In [ ]: 
plot(V[:,1], V[:,2], label="Напряжение, В", linewidth=2, title="Напряжение LC-осциллятора")
Out[0]:
In [ ]: 
plot(V[:,1], V[:,2], label="Напряжение, В", linewidth=2, xlim=(4e-5, 4.1e-5), title="Напряжение LC-осциллятора в течение 1 микросекунды")
Out[0]:

Blocks used in example